A Mathematical Appeal to Wear a Mask

Photo Credit: Vera Davidova

Courtesy of Unsplash

While many people have adjusted into a new normal in light of the COVID-19 pandemic, some are easing back into recreational activities and returning to their pre-pandemic lifestyles. For those of you who stay near campus, you might have noticed evenings becoming increasingly chaotic. However, what struck me most was a Snapchat video showing hundreds of students at a party on Pearl Street without masks or any type of social distancing. As COVID-19 cases continue to spike, I see an increasing need to emphasize the importance of wearing masks in indoor spaces, especially with Thanksgiving and Christmas coming up soon. With this in mind, I have generated a comprehensive mathematical model to demonstrate the effectiveness of wearing a mask in public.

Let’s imagine there are two students in a room, Dan and Amanda. Amanda has contracted COVID-19 while Dan is COVID free. Amanda releases a single virus particle into the room. We can call the probability per minute that Dan will interact with the virus, β , and therefore the probability of that person not interacting with the virus is 1 – β per minute. This is because, given any probability p for an event, the probability that the event doesn’t occur is 1 – p. In t minutes, this equation becomes (1 − β)^t . We raise this probability to t because in one minute, the probability is (1 − β)^1 , in two minutes, (1 − β)^2 , and etc. Using the 1 – p rule again, the probability of that person interacting with the virus in t minutes can now be expressed as following:

Equation 1.

Now, I’ll expand this equation to consider the scenario where Amanda releases more than one virus particle in the room. We can imagine that Amanda releases a certain number of virus particles per minute, or α. In t minutes, she will then release a total of α * t virus particles. The probability of Dan interacting with any of the α * t virus particles in t minutes can be expressed as following:

Equation 2.

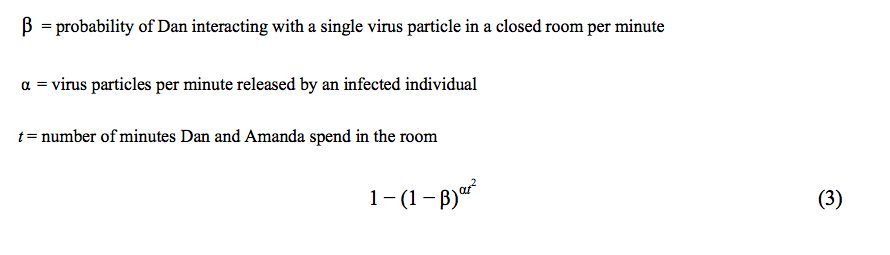
Finally, by replacing q with our result from equation 1, we have the probability of Dan interacting with the virus from an infected individual, where α is the virus-releasing rate, and β is the probability of Dan interacting with the virus in a closed room as:

Equation 3.

Now, let’s explore the scenario where Amanda wears a face mask with a filtering rate of f . This is the probability that the face mask will filter out the virus. For reference , standard blue surgical masks provide a filtering rate of 45% – 55%. Since Amanda is wearing a mask, the rate at which she releases the virus becomes α (1 − f ) , where 1 − f is the probability that the mask will not filter out the virus. Thus, allowing the equation to be modified into the following form:

Equation 4.

Now, if Dan also wears a face mask with a filtering rate of f , then the probability of him interacting with the virus becomes:

Equation 5.

From the equation derived above, we could conclude that if the infected individual and the healthy person both fail to wear face masks, then the probability of interacting with the virus f increases quadratically with respect to time. To better visualize this, let us consider two scenarios. First, assume Dan and Amanda are in a room. Amanda has contracted COVID-19 while Dan has not and neither of them are wearing masks. We will assume Amanda releases virus particles at a rate of 20 particles per second and the probability of Dan interacting with a single virus in the room is 2% per minute. Using equation 3:

Graphing this function:

Now consider the same scenario except both Amanda and Dan are wearing masks with a filtering rate of 50%. Using equation 5:

Graphing this function:

Now, comparing the graphs of these two functions:

In this scenario, without masks, Dan would have about an 80% chance of interacting with a virus particle from Amanda after two minutes in the room. Compare this to a situation where Dan and Amanda are both wearing masks. In this case, Dan only has about a 32% chance of interacting with a virus particle after two minutes in the room. In general, if the infected individual wears a mask, then every healthy person in the room is protected by a rate of f. Similarly, for any healthy person who wears a face mask, they are also protected by a rate of f . More importantly, if both infected and healthy people wear face masks, the possibility of contracting the virus is drastically reduced. See this figure from the Lincoln Journal Star, explicitly showing how masks effectively reduce the transmission of the virus. Note that the diagram uses different flow rates for the virus flowing in and out of the mask while we assumed both rates to be equivalent in the models above. Also, see figure 6 of this study, in which a research group from the University of Nicosia in Cyprus maps out the expected flow patterns of small droplets released when a person coughs. The flow patterns are markedly higher while not wearing a mask, in contrast to a lower flow pattern while wearing a mask.

It is important to note a few limitations of this model. As mentioned, this model assumes the filtering rate for viruses flowing in and out of the mask are the same and that Dan and Amanda wear masks with the same filtering rate. These assumptions are made for the sake of simplicity and, even with differing rates, a similar effect would be observed. This model also models virus interaction, not infection. This too is done for the sake of simplicity and reasonably assumes that reducing virus interaction reduces infection. Now, while mask wearing is a critical step in protecting yourself and others from COVID infection, it is no panacea. Other precautions, such as social distancing and quarantining , are important steps you should take to ensure the safety of those around you.

For those who have decided to attend gatherings, it is critical to take precautions to protect yourself and others. Regardless of how safe the activities may feel, the risk of exposure and the probability of contracting COVID-19 is still a risk. However, by simply putting on a mask, you could significantly help minimize the spread of the virus, helping to protect yourself and your community.